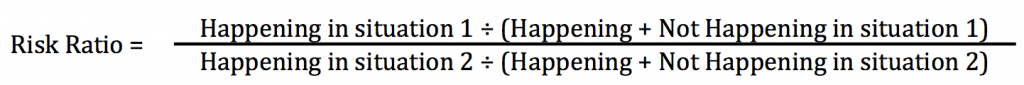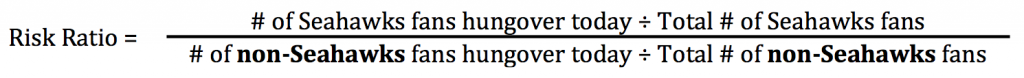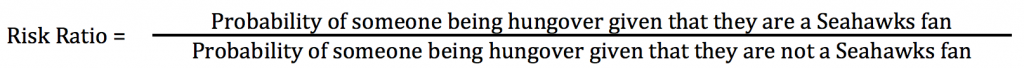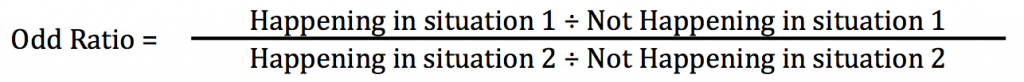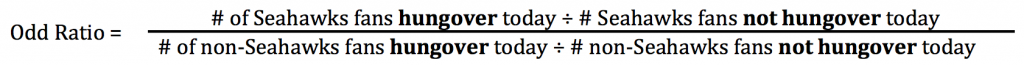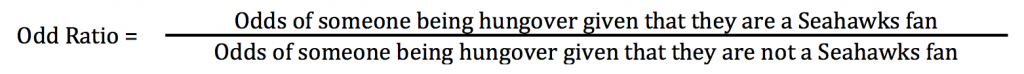Odds, Probability, Chance, Risks: Interchangeable?Not so much.
What does it mean to say “smokers are X times more likely to get lung cancer than non-smokers?” What about when the weather channel says, “there is a 10% chance of rain?” The odds of 1 to 10 of winning?
These words are often used in casual conversations as somewhat interchangeable, and can be rather confusing. I remember being very excited to learn about them for the first time, so hopefully you will find this as interesting (or at least as clarifying!) as I did!
A little test!
In which of the following scenario are you most likely to find dessert happiness?
Which ones are saying the same thing?
A. The odds against you eating a cupcake are 1 to 5.
B. Your odds of/on eating a cupcake are 1 to 5.
C. The probability of you eating a cupcake is 20%.
D. You have a 20% chance of eating a cupcake.
Answers, in short: A is the most likely-for-cupcake scenario, and C and D are saying the same thing.
Click here for the long answer!
The differences between odds and probability lies in their ranges and denominators.
Ranges
Odds are bound between 0 and positive infinity.
That is, you can have the odds of 0:100 (not gonna happen), or 10000:1 (10-thousand times more likely to happen than not). There are no negative odds.
Probability is bound between 0 and 1.
That is, when probability = 0, an event is impossible; when probability =1, it is definite. There is no negative probability, and no 120% probability of happening (sorry!).
Denominators in formulae
Odds: Happening ÷ Not Happening
Probability: Happening ÷ (Happening + Not Happening)
Note
Note that the odds for X and odds against X are reciprocals of each other (1/3 is the reciprocal for 3/1), whereas for probabilities, the probability of X and the probability of not X are complements of each other. (Thank you, Jeff K. Bye, for contributing this note for clarification!)
For example, there are 4 cats in the hat. 1 is orange, and 3 are brown.
Odds of Orange Cats
1 orange to 3 brown. This can be written as 1:3, or 1/3, or 1-3
“The odds of/on pulling an orange cat out of the hat are 1 to 3.”
“The odds against pulling an orange cat are 3 to 1.”
Probability of Orange Cats [often written as P(Orange Cat)]
1 orange ÷ (1 orange + 3 brown) = 1 orange ÷ 4 total cats = 1/4 = .25 = 25%
“The probability of pulling an orange cat out of the hat is 25%.”
or “There is a 25% chance of pulling an orange cat out of the hat.”
or “There is a 1 in 4 chance of pulling an orange cat out of the hat.”
Converting between odds and probability
Converting Odds to Probability:
Simply add the 2 components of the odds together to make a new denominator, and use the old numerator.
e.g. If the odds are 3:5, or 3 to 5, the probability is 3 ÷ (3+5) = 3/8 = 37.5%
Converting Probability to Odds:
Take the probability, and divide it by its compliment = (1-itself).
e.g. If the probability is .4, or 40%, the odds are .4 ÷ (1-.4) = .4 ÷.6 = 67%, or 4:6.
Taking a little Risks in Probability: Risk Ratios
When they say things like “smokers are X times more likely to get lung cancer than non-smokers,” they are utilising Risk Ratios.
Risk ratio is the ratio of two probabilities:
For example, the relative risk of being hungover today, associated with being a Seahawks fan would be
Which is equivalent to
Risk Ratio is often expressed as a factor and a whole positive number, such as “… is 20 times more likely…”
The difference between odds ratio and risk ratio
While Risk Ratio is the probability of one thing divided by the probability of another (usually in a separated group), Odds Ratio is the odds of one event happening divided by the odds of another.
Back to the Superbowl example, the relative odds of being hungover today, associated with being a Seahawks fan would be
In other words, this is
Test, revisited!
A. The odds against you eating a cupcake are 1 to 5.
These are your best odds (5:1)! You are 5 times more likely to eat cupcake than not!
P(cupcake) = 5 ÷ (5+1) = 5/6 = 83.3%
B. Your odds of/on eating a cupcake are 1 to 5.
Your odds are much sadder. Though interesting thing is, that B and A adds up to 100%, because they are counterparts to a whole.
P(cupcake) = 1 ÷ (5+1) = 1/6 = 16.7%
C. The probability of you eating a cupcake is 20%.
Odds are .2/(1-.2) = .2/.8 = .25, or 1:4.
Still better odds than B.
D. You have a 20% chance of eating a cupcake.
This (most likely*) says the same thing as C.
*This is the caveat. Sometimes people say chance interchangeably with odds, also. The chance (ha!) of this occurring is very rare in scientific literature though. It is most often use in casual conversation to imply an imprecise probability, as the previous sentence demonstrated!
Acknowledgements
Thank you, Jordan Essoe, for helping brainstorm the title. Thank you, Jordan and Joshua Essoe, for helping me discern which of terminology are un/familiar to the public. Thank you, Jeff K. Bye, for statistical advises!